Dynamic Calculus
Home > Introduction
The calculus controversy
Image 1

Transcript of audio file: Calculus is sometimes referred to as the 'mathematics of change'. It is the branch of mathematics concerned with describing the precise way in which changes in one variable relate to changes in another. Of particular importance to many fields of human endeavour is its capacity to compare quantities which vary in a non-linear way. As such, calculus is a vital tool in many fields of study in science and engineering.
Image 2
Transcript of audio file: Calculus has been described as one of the major intellectual achievements of Western civilization. Practical applications of calculus in our modern world include areas as diverse as computer science, astronomy, meteorology, oceanography, statistics, economics, business, sociology, medicine, architecture and aviation.
Image 3
Transcript of audio file: There are two main, interconnected branches of calculus. The first is differential calculus, which is the study of how functions change when their inputs change. At its core is the process of ‘differentiation’, which allows us to find the gradient of a tangent to a curve at a point.
Image 4
Transcript of audio file: The second branch is integral calculus. The operation known as ‘integration’ is also referred to as the process of ‘anti-differentiation’. It relates to the accumulation of quantities such as the area of a region under a curve.
Image 5
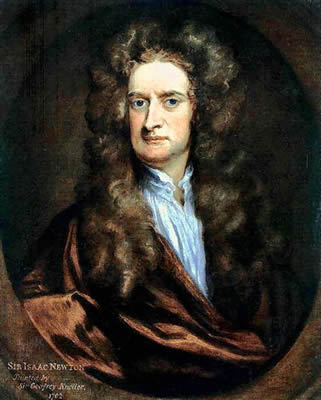
Transcript of audio file: As is the case with many discoveries, the development of calculus was the culmination of centuries of work by many esteemed mathematicians. Towards the end of the 17th century, the Englishman Sir Isaac Newton and the German Gottfried Leibniz were both working independently on problems of motion.
Image 6

Transcript of audio file: Today, there is a general consensus that credits both with the development of calculus. At the time, however, a bitter and divisive dispute as to who first invented the calculus raged in academic circles.
Image 7

Transcript of audio file: Newton claimed to have begun work on an early form of calculus in 1666, which he called ‘the method of fluxions and fluents’. Much of the ensuing controversy resulted from the fact that Newton did not publish this work until decades later.
Image 8

Transcript of audio file: Leibniz began working on his version of the calculus in 1674. In 1684 he published his first paper on differential calculus and then published his explanation of the integral calculus in 1686. On a visit to London in 1676, Leibniz was reportedly shown at least one unpublished manuscript by Newton. It was later claimed by Newton and his supporters that Leibniz had merely invented an alternative notation for ideas that were first developed by Newton.
Image 9

Transcript of audio file: Claims and counter-claims of plagiarism escalated the dispute and in the years before his death in 1716, Leibniz was embroiled in a bitter defence of his work. Whilst Leibniz admitted to being shown the early work of Newton, his private papers demonstrate that his development of the calculus was quite different from the approach taken by Newton.
Image 10

Transcript of audio file: In what had become an issue of English national pride, in 1715 the Royal Society conducted an investigation into the matter and perhaps, not surprisingly, credited Sir Isaac Newton with the discovery of the calculus. Modern opinion, however, recognises the great contributions made by both men and they are now generally given credit for jointly and independently developing this powerful branch of mathematics.